Finding some wiggle room in semiconductor quantum computers
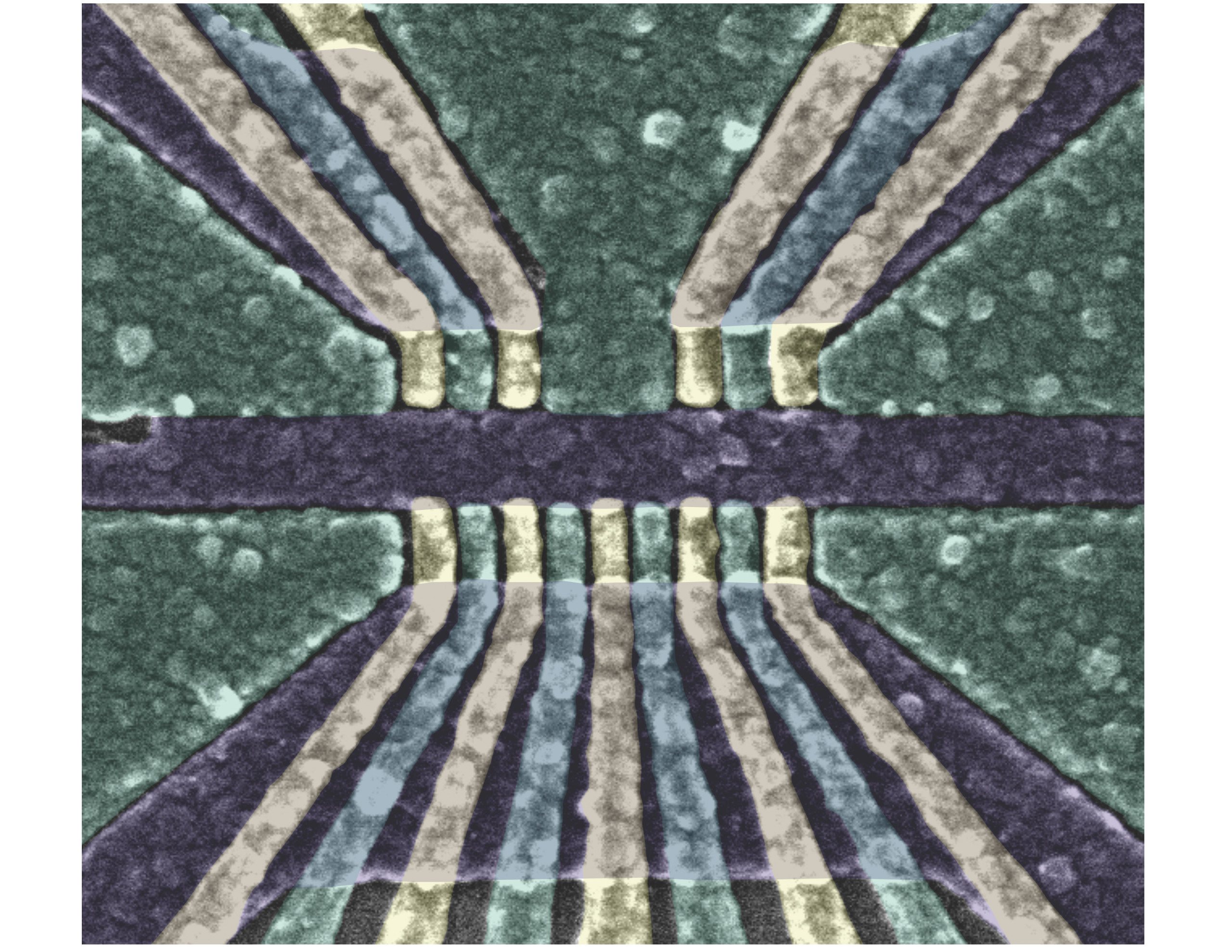
A false-colored scanning electron micrograph of the qubit structure featuring the “wiggle wells” developed by researchers at UW–Madison to improve the accuracy of quantum computers. The imaged area is about 1,500 nanometers across. For comparison, a human hair is between 50,000 and 100,000 nanometers wide. University of Wisconsin–Madison
Classical computers rarely make mistakes, thanks largely to the digital behavior of semiconductor transistors. They are either on or they’re off, corresponding to the ones and zeros of classical bits.
On the other hand, quantum bits, or qubits, can equal zero, one or an arbitrary mixture of the two, allowing quantum computers to solve certain calculations that exceed the capacity of any classical computer. One complication with qubits, however, is that they can occupy energy levels outside the computational one and zero. If those additional levels are too close to one or zero, errors are more likely to occur.

Mark Friesen
“In a classical computer, all the aspects of a transistor are super uniform,” says UW–Madison Distinguished Scientist Mark Friesen, an author on both papers. “Silicon qubits are in many ways like transistors, and we’ve gotten to the stage where we can control the qubit properties very well, except for one.”
That one property, known as the valley splitting, is the buffer between the computational one-zero energy levels and the additional energy levels, helping to reduce quantum computing errors.
In two papers published in Nature Communications in December, researchers from the University of Wisconsin–Madison, the University of New South Wales and TU-Delft showed that tweaking a qubit’s physical structure, known as a silicon quantum dot, creates sufficient valley splitting to reduce computing errors. The findings turn conventional wisdom on its head by showing that a less perfect silicon quantum dot can be beneficial.
In the past, the most common way to make qubits was to embed the quantum dot in a layer of pure silicon, called a silicon well, and then sandwich that layer between two layers of silicon-germanium with sharp boundaries between the layers. In these new studies, the semiconductor qubits are made similarly, with a silicon layer sitting between two layers of silicon-germanium. The new feature is the addition of germanium to the silicon layer itself.

Bob Joynt
“Everyone always thought that the one thing you shouldn’t mess with in the qubit design is the pure silicon,” says UW–Madison physics professor Bob Joynt. “And we decided, well, let’s just mess with it a little bit.”
The researchers messed with the design by intentionally adding germanium to the silicon layer in slightly different ways. Like so many successes in physics, theirs started with theoretical calculations. Joynt asked what would happen if the germanium concentration ‘wiggled’ throughout the well, in regularly spaced waves with peaks and valleys. Friesen’s group, which also contributed to the companion paper from TU-Delft, noted that a little germanium always spills into the well even when researchers try to keep it out. They asked what would happen if low levels of germanium were sprinkled randomly throughout the well.
“The theory calculations showed unambiguously that it’s better to include germanium, and it’s better to wiggle than not to wiggle,” says UW–Madison physics professor Mark Eriksson, whose group tested the new wiggle well qubits.

Mark Eriksson
After confirming that the wiggle wells did not significantly change the quantum dot’s electronic properties, Eriksson’s group measured the size of the energy buffer, or valley splitting, in these new structures. For a quantum dot embedded in a wiggle well, the theory predicted a 20 microelectronvolt increase. But in the lab, the largest valley splitting measured was nearly 250 microelectronvolts. Further, the valley splitting changed when the quantum dot was moved to new locations in the well, where the atoms making up the wiggles were in different locations. The wiggle in the concentration was clearly not the only factor affecting valley splitting — the individual atoms mattered too.
This fact turned out to be key: the atoms in the wiggle well were distributed somewhat randomly. Introducing this random distribution into numerical simulations accurately explained the observed valley splitting variations. In the second paper, Friesen and his TU-Delft collaborators confirmed that even uniformly distributed, random germanium in the well leads to larger valley splitting on average than when no germanium is present.
“Contrary to many people’s fears, germanium in the wells is a really good idea,” Eriksson says. “If you sprinkle it completely randomly with no concentration oscillations, you’re going to do pretty well. If you sprinkle it with concentration oscillations, you’ll do better.”
Both qubit structures have been filed for patent protection by WARF, and the UW–Madison team is actively working on designs that further enhance the valley splitting.
“It is possible that when quantum computers are made from silicon/silicon-germanium qubits, they will want to have germanium in the wells,” Joynt says. “But we don’t know yet. We’re just getting started.”
At UW–Madison, Thomas McJunkin, Benjamin Harpt, Yi Feng, Merritt Losert, J. P. Dodson, Michael Wolfe, Don Savage, Max Lagally and Sue Coppersmith additionally contributed to this work. Both studies were funded by the Army Research Office, a directorate of the U.S. Army Combat Capabilities Development Command Army Research Laboratory (W911NF-17-1-0274).
Tags: computer science, physics



